Elongated triangular bipyramid
| Elongated triangular bipyramid | |
|---|---|
| Type | Johnson J13 - J14 - J15 |
| Faces | 6 triangles 3 squares |
| Edges | 15 |
| Vertices | 8 |
| Vertex configuration | 2(33) 6(32.42) |
| Symmetry group | D3h |
| Dual polyhedron | Triangular bifrustum |
| Properties | convex |
| Net | |
In geometry, the elongated triangular bipyramid (or dipyramid) is one of the Johnson solids (J14), convex polyhedra whose faces are regular polygons. As the name suggests, it can be constructed by elongating a triangular bipyramid (J12) by inserting a triangular prism between its congruent halves.
The nirrosula, an African musical instrument woven out of strips of plant leaves, is made in the form of a series of elongated bipyramids with non-equilateral triangles as the faces of their end caps.[1]
Contents |
Formulae
The following formulae for volume and surface area can be used if all faces are regular, with edge length a:[2]
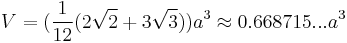
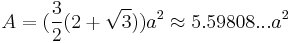
Dual polyhedron
The dual of the elongated triangular bipyramid has 8 faces: 6 trapezoidal and 2 triangular.
| Dual elongated triangular bipyramid | Net of dual |
|---|---|
References
- ^ Gerdes, Paulus (2009), "Exploration of technologies, emerging from African cultural practices, in mathematics (teacher) education", ZDM, the International Journal on Mathematics Education 42 (1): 11–17, doi:10.1007/s11858-009-0208-2.
- ^ Stephen Wolfram, "Elongated triangular dipyramid" from Wolfram Alpha. Retrieved July 22, 2010.